Summary of a draft Research Article – Category: Atmosphere – Guest contribution of Frans van den Beemt – Physics of CO2 absorption processes: an original upper limit calculation of infrared CO2 absorption in case of a doubling of CO2 in the earth atmosphere
Frans van den Beemt
Abstract
We analyzed CO2 absorption and emission of infrared radiation and calculated the upper limit of a possible temperature increase at the earth’s surface, including the effect of average annual cloud cover. The calculations are based on measured spectra data of CO2 and we computed those using the general laws of physics and quantum mechanics. We found 1.33 W/m2 extra absorption for doubling CO2 of which we assume that half will be lost into space and half (0.67W/m2 ) contributes to a surface temperature increase with a worst case upper limit of 0.16K. We assess that the assumptions in IPCC report 2015 of 3.8W/m2 and 1K increase respectively is overestimated considerably
Samenvatting
We analyserden de CO2-absorptie en emissie van infraroodstraling en we berekenden de bovengrens van een mogelijke temperatuurstijging van het aardoppervlak, gebruik makend van de algemene wetten van de natuurkunde en de kwantummechanica. We vonden 1.33 W/m2 extra absorptie voor verdubbeling van CO2 waarvan we aannemen dat de ene helft verloren gaat in de ruimte. De andere helft (0.67W/m2) draagt bij aan een temperatuursverhoging met een worst-case-bovengrens van 0.16K. We concluderen dat de IPCC-aanname van 3.8W/m2 [IPCC-rapport 2015] en 1K toename in oppervlaktetemperatuur (zonder feedbacks) een aanzienlijk overschatting is.
Introduction
The temperature of the earth atmosphere depends on several physical processes, to mention some important ones: water -evaporation, -condensation and -crystal formation, cloud formation, aerosols, convection, wind, gravitation, earth rotation and the radiation properties of its Infrared (IR) gasses. The question addressed here is, how an increase in Carbon Dioxide concentration will affect the earth atmosphere temperature at the surface.
Infrared active gasses (like H2O, CH4, CO2) in our atmosphere interact with IR emissions of the earth surface. In this article we will focus on the physics of this interaction for Carbon Dioxide (CO2) only. CO2 molecules absorb IR radiation from the surface and via collisions this energy will be transferred to all the neighboring molecules in the local atmosphere. The further transport of this energy happens mainly through transport of molecules in the never resting atmosphere, by wind advection and convection. Part of the IR emissions from the surface as well as from the atmosphere and clouds will be lost to space and this cools the earth and atmosphere.
Methods
We refrain from using the most common approach to analyse CO2 concentration and its effect on atmospheric temperature, which is using numeric methods based on well formulated radiation transport equations throughout the atmosphere. Instead, we take a different and overarching approach. First, we calculate an upper limit of absorption of energy (additional absorbed energy) by CO2 in the atmosphere for a doubling of the CO2 concentration. Next, we use this additional energy to calculate a best guess for a temperature rise, taking into consideration a global yearly average cloud cover due to convection and advection processes. The cloud cover shields incoming radiation from the sun and outgoing IR radiation from the surface and atmosphere. The IR emissions of cloud cover are assumed to contribute to emissions to space as well as emissions to the surface. In this paper we do not elaborate on IR radiation by clouds as clouds already absorb all IR radiation emitted from the surface beneath it, regardless of CO2 concentration. Hence, doubling of CO2 concentration has no effect on the amount of extra CO2 absorbed energy under cloud cover. Consequently doubling of CO2 concentration can only have effect on the clear sky parts of the atmosphere and this is calculated herein.
As we are searching for the maximum possible absorption by CO2 molecules, we have no interest in radiation transport as such. Therefore, we will not need to use radiation transport equations and associated correction factors. Energy transport throughout the atmosphere is a given and we use its most important result, namely cloud cover.
The CO2 667 cm-1 (15µm) spectral line is the most important in CO2 absorption, radiation and heat transfer in the Earth atmosphere. In particular its vibration V2 energy level together with its vibration-rotation lines (fig 2, source Purdue)
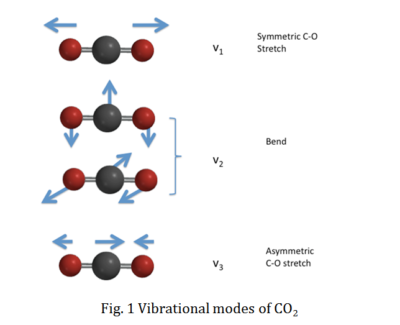Our calculations are based on the theory and formulas derived from Beer–Lambert law, Kirchhoff’s law, Planck’s law, Boltzmann distribution named after Ludwig Boltzmann and Stefan–Boltzmann law and Einstein coefficients for emission and absorption. We also use the measured CO2 spectra data from the HITRAN database [Rothman 2004][i].
CO2 has a central band or branch Q and side bands, brancha or ‘wings’ P and R with spectral lines, determined by quantum rotational numbers J, see Figure 2 [Harde 2013] [ii]. Quantum rotational numbers are used to distinguish each separate energy level for each unique frequency or wavenumber.
We use calculations quantifying the IR absorption by CO2 molecules in the atmosphere, which originate from the Earth surface with a temperature Ts. We assume black body radiation from the surface. We calculate the CO2 absorption in a straightforward manner, especially in its far side wings, which are not saturated and will absorb some more radiation with increasing CO2 concentration. The center band Q and part of the side wings are already saturated at 300ppm CO2. The additional absorption comes from the far side wings. For the P wing we calculate the absorption contribution for the rotational-vibration lines for J=44 to J=70 and for the R wing J=46 to J=66. These far side wings absorb throughout the troposphere.
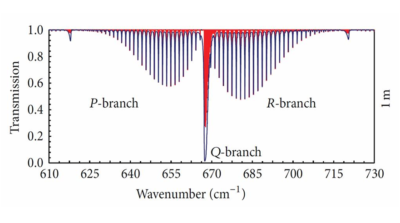
Figure 2: Absorption spectra for 380 ppm of CO2 in dry air at 1m propagation length (air pressure: 1013 hPa, temperature: 288K). Vibration lines with superposition of several fine rotation lines [H. Harde 2013;page 20 Figure 13]ii. Within one meter all IR radiation with a wavenumber close to 667 cm-1 will be absorbed or in other words the transmission becomes zero for these wavenumbers after 1 meter traveling in the atmosphere.
We complete this analysis with a calculation of the expected temperature rise of the surface due to additional (far side wings) absorption of radiation by CO2 molecules in the atmosphere, when doubling the CO2 concentration from 300 to 600 ppm (parts per million). In each step of our calculation we ensure using values, which guarantee an upper level calculation as an estimation of the worst case scenario. For instance, using Ts=288K and 1 atmosphere on the whole path length from surface up all the way to the top of the troposphere. This ensures much more absorption than in reality with declining temperatures and pressures at altitude (and thus fewer collisions). A ballpark calculation shows an overestimation of the extra absorption of about a factor three for which we did not correct. At doubling of CO2 concentration from 300ppm to 600ppm we have calculated an additional absorption of 3.5W/m2 under clear sky conditions only, for reasons mentioned earlier. The global / yearly average clear sky area is reported to be 38% [iii]
That leaves us with 1.33 w/m2 extra absorption of which we assume that half will be lost into space and half (0,66W/m2 ) contributes to a temperature increase with an worst case upper limit of 0,16K. so in reality will be much less. The IPCC reports an additional absorption of 3.8W/m2 [IPCC report 2015] that contributes to an increase of the surface temperature of 1K, without additional (H2O) forcing. [H. Harde 2013]ii found 2.6W/m2 and 0.3K (for CO2 doubling from 380ppm to 760ppm), [H. Hug 2012]iv found 0.2K (for CO2 doubling from 200ppm to 400ppm).
The models of IPCC use a H2O vapor gain factor due to positive feedback. IPCC assumes that, after increasing the temperature near and at the surface, more water vapor will enter the atmosphere and as a consequence, more absorption by H2O molecules will occur followed by a further increase of temperature at the Earth surface. But as is the case with CO2, also water vapor spectral lines are already saturated and no additional absorption will take place. Evaporation of water takes a substantial amount of energy, which is no longer available for a temperature rise at the surface. This way water molecules function as a thermostat for the earth surface temperature. Furthermore, H2O vapor spectra overlap the spectra of the collision broadening lines of CO2. Hence, the influence of CO2 on additional absorption decreases. Therefore, we assess that the results as reported by IPCC show an unrealistic high increase of temperature.
Footnotes and References
[i] Rothman, L. S. et al, (30 authors), J. Quant. Spectrosc. Rad. Trans. 96 139-204 (2005), ‘The HITRAN 2004 molecular spectroscopic database’
[ii] Herman Harde Radiation and Heat Transfer in the Atmosphere: A Comprehensive Approach on a Molecular Basis, Hindawi —Publishing Corporation International Journal of Atmospheric Sciences, Volume 2013, Article ID 503727, 26 pages (2013) http://dx.doi.org/10.1155/2013/503727 [H. Harde 2013]
[iii] [ J.T. Kiehl and Kevin E. Trenberth, Earth’s Annual Global Mean Energy Budget, Bulletin of the American Meteorological Society Vol. 78, No. 2, February 1997].
[iv] Heinz Hug, Der anthropogene Treibhauseffekt – eine spektroskopische Geringfügigkeit , Wiesbaden (August 2012) https://www.eike-klima-energie.eu/wp-content/uploads/2016/12/Hug-pdf-12-Sept-2012.pdf [H. Hug 2012]
About Frans van den Beemt
Frans obtained his MSc physics in June 1973 at the University of Technology, Eindhoven, The Netherlands and his PhD in Science Studies, June 2000, University of Leiden, The Netherlands
He held positions as Teaching Assistant, University of Technology, Eindhoven, The Netherlands, then as Research Fellow, Department of Experimental Nuclear Physics, University of Technology, Eindhoven, The Netherlands. Next he was teacher in Physics and Mathematics, Eckhart College, Eindhoven, The Netherlands, System Engineer, Machines & System Group Holec, Ridderkerk, The Netherlands and Program Director, Technology Foundation STW, Utrecht, The Netherlands He is founder of consulting firm: VdBeemt 2G Advies and founder of European academia consulting firm: HandsonGrants. He authored numurous publications and performed many specialized functions.
Erratum: corrected typo h/t Ronald
Erratum2: corrections corrected: We found 1.33 W/m2 extra absorption for doubling CO2 of which we assume that half will be lost into space and half (0.67 W/m2 ) contributes to a surface temperature increase with a worst case upper limit of 0.16K.
Hi Frans,
IPCC 3.7 W/m2 is based on Myhre et al 1998, who derived F=c• ln(C/ C0) , with c= 5.35
Myhre, G., Highwood, E. J., Shine, K. P. and Stordal, F. (1998) New estimates of radiative forcing due to well mixed greenhouse gases. Geophysical Research Letters, 25, 2715-2718.
https://agupubs.onlinelibrary.wiley.com/doi/pdf/10.1029/98GL01908
Did you adjust for pressure broadening in the spectral bands? That does increase the absorption dramatically.
A quote from the draft main article
It’s in your mailbox.
Due to technical problems, relaying the answer of the Author, Frans van den Beemt
Recently the value for co2 doubling was raised from 3.7 W/m2 to 3.8 W/m2. This value was also used by Lewis and Curry 2018 for ther climate sensitivity estimates.
See: https://niclewis.files.wordpress.com/2018/04/lewis_and_curry_jcli-d-17-0667_accepted.pdf ; section 3a
H/t Frank Bosse at Judith Curry’s blog.
This article had more than a few problems. 1. It does not recognize that CO2 and H2O absorb infrared at different frequencies, 2. It claims that half of absorbed infrared is emitted to space despite zero infrared detected from Earth at those frequencies
Paul Excuses for the formatting problems in this post.
(1) Could you please quote exactly where the article claims or implies not to recognize that CO2 and H2O absorb infrared at different frequencies?
(2) obviously this statement is too ambiguous:
It’s intended to indicate that all absorbed energy will be emitted eventually in a dynamic equilibrium. And from escaping from the atmosphere there are two options, up or down, it either disappears into space or it hits the earth surface, known as back radiation. So could you point out any error in this logic?
energy does not “disappear”, since it can’t neither be created nor destroyed….
Sorry for the ambiguity but when the energy “disappears into space” it is merely emitted away from earth. It is not destroyed and it still exists elsewhere, receding with the speed of light and it’s no longer available on Earth.
That is right.
Reply of the author:
For weather considerations, processes in the atmosphere are highly relevant. But, when one looks down in a swimming pool, one clearly sees the bottom. I.e. light travels down into the ocean. It is only gradually absorbed. And it takes ~ 100+ m before it is almost 100% transferred to the water. The energy of the sun is thus partly transferred tot water at places where even the diurnal cycle is inadequate to describe the total proces of absorption and re-emission. This must be relevant to climate, or rather the weather over an anual cycle.
To what extend is this proces of a substantial amount of incoming solar energy that is stored in the heat battery of the oceans influencing the heat (energy) transfer and therefore the temperature of which we talk, when dealing with the almost negligable heat content of the atmosphere at the boundary ocean/solid ground vs. air?
Pictures of the earth seen from space do not show a black body with radiation only lacking a few narrow bands belonging to CO2, NO2, H2O etc. I am afraid we are missing here something.
Kees,
The atmosphere is opaque for IR apart from as you stated a narrow band.(1). A large amount of energy is transported by means of the H2O cycle. Evaporated sea water is transported (via Hadley cells) as moist air to higher altitudes (and forced at the end to the poles). The heat ends therefore also partly at the TOA via (wet) convection. Meteorologists know this already long time ago. Climate science is very preoccupied with CO2 as the thermostat, while Frans (see also (2)) states in his article that H2O acts actually as the thermostat.
Varying heat storage in sea heat battery is responsible for varying temperature (e.g. ENSO). What we are “missing” is the status of science pre-Hansen. Let’s go back to the status of 1985 and starts again taken into account not only the radiation balance influenced by CO2 and H2O, but also other components like clouds and energy from the heat bath of sea and its transport to TOA. Climate is too complex that you can simplify it by a single atmospheric parameter as CO2 (which is actually hardly doing anything)
(1) IR opaque for 68 % for clear sky, private communication Frans van der Beemt.
(2) https://www.arthurrorsch.com/
Isn’t the energy content of the solar radiation of shorter wavelength then infrared substantial? That part of the insolation reaches the oceans. Part is reflected the remainder is absorbed at distance from the surface. The accumulating energy must get out again in a diurnal and even anual cycle.. (Temperatures within a turbid medium can be quite a lot higher than at the surface of an opaque medium with same emissivity (epsilon) in radiative equilibrium.) The out flux is mostly by latent heat, I suppose. Another part by conduction to the air and by Stefan-Boltzman radiation from the surface. There is also radiation from the oceans coming from layers below the surface.The heat conduction of water is low, convection however does transport energy efficiently. Evaporation is subject to transport of water vapour away from the surface (wind and convection). There is diffusion and conduction also, but they are rather small. All in all the system is very complex, much so that my attempts to start modelling and calculation have failed thus far. But the complex must be very influential to climate, less to weather, strange as it sounds. That is because in the diurnal cycle – I am tempted to say ‘Arthur’s cycle’ – we see (measure) only small variations in temperature of the water surface over day and night in the oceans.
Indeed Arthur’s cycle of the diurnal one is far too short to model climate changes. As you mention you need data at a seasonal or multi-year (planetary) scale, although the basic principle that the heat has to be removed within any cycle is still valid (an autonomous system).
That your attempts to model failed so far I am not surprised. One needs detailed information about sea currents, wind and evaporation. Too complex and lack of proper detailed data. The computer says “insufficient data”.
Reply of the author:
Arthur Rörsch presents a calculation in his “rethinking” and concludes a radiative forcing of 3.6-4.1 W/m2, in agreement with the number presented by IPCC, i.e., 3.7W/m2.
Reply of the author:
The problem with the observation of Heinz Hug is that his observation does not stretch out far enough into the wings of the absorption band, that is why he was measuring saturation.
Absorbance
http://members.casema.nl/errenwijlens/co2/Hug_absorbance.gif
Absorption
http://members.casema.nl/errenwijlens/co2/hug_absorption.gif
Reply of the author:
I’m sorry Frans that is simply not true: Heinz did send me his data and it is is clear that he did not extend his measurements into the partly saturated wings.
Please have also a look at my own CO2 absorption calculations, with downloadable spreadsheet.
https://klimaathype.wordpress.com/2015/12/14/over-de-verzadiging-van-het-co2-spectrum/
Another issue to consider is that the vertical temperature profile governs the amount of absorbed energy. In the case of temperature inversion as is the case in Siberian and Antarctic winter, the co2 absorption band even changes into an emission band and adding co2 will increase emission and so cool the lower layers.
http://1.bp.blogspot.com/-PwdZCD2eZ6Y/UOVpiq1CrKI/AAAAAAAAPng/Lp-Ue4Xiuuo/s1600/atmospherespectra.gif
Which temperature profile was chosen in your study?
Reply of the author
Your assertion that “Evaporation of water takes a substantial amount of energy, which is no longer available for a temperature rise at the surface” is not substantiated and may be erroneous since it is neither fully known nor fully understood what the net effect of additional water vapor, due to the temperature increase on account of doubling the CO2, on the temperature of the biosphere is in general and the earth’s surface in particular.
Keep in mind that water evaporation can eventually result in an increase in cloud coverage and hence can result in a cooling effect. Additionally, note that the water evaporated will eventually condense and thus release a substantial amount of energy which may then be available for a temperature rise of the atmosphere, thus enhancing heat losses into outer space and heat gains at the surface by radiation…..
It is not clear why the effect of overpopulation on the biosphere is not addressed. After all, Your assertion that “Evaporation of water takes a substantial amount of energy, which is no longer available for a temperature rise at the surface” is not substantiated and may be erroneous since it is neither fully known nor fully understood what the net effect of additional water vapor, due to the temperature increase on account of doubling the CO2, on the temperature of the biosphere is in general and the earth’s surface in particular.
Keep in mind that water evaporation can eventually result in an increase in cloud coverage and hence can result in a cooling effect. Additionally, note that the water evaporated will eventually condense and thus release a substantial amount of energy which may then be available for a temperature rise of the atmosphere, thus enhancing heat losses into outer space and heat gains at the surface by radiation…..
It is not clear why the effect of overpopulation on the biosphere is not addressed. After all, it is us humans who are poisoning the environment, even though CO2 is not a polliutant…..
Ben
Let’s substantiate “Evaporation of water takes a substantial amount of energy, which is no longer available for a temperature rise at the surface”
https://www.engineeringtoolbox.com/steam-vapor-enthalpy-d_160.html
For every 1 meter of water evaporating at the surface per square meter per year makes 0.03 g per second * 22 translates to a flux of 71.5 W/m2.
For 15 degrees C an equilibrium absorbed flux is 390 W/m2 (Stefan Boltzman). So if 71,5 W/m2 thereof is used for evaporation the remaining 318.5 W/m2 is only good for 274K or 1 degrees C. That’s how evaporation removes energy that is no longer available for a temperature rise at the surface.
Since you said “For every 1 meter of water evaporating at the surface per square meter….”, then, your conclusion should then be “the remaining 318.5 W/m2 is only good for 274K or 1 degrees C per meter of water evaporated.”: this is rather meaningless since it is not known hopw much water evaporated.
Any way, it seems that you missed my point: the water evaporated eventually will condense and release its heat of vaporization into the atmosphere resulting in a warming of the surrounding atmosphere surrounding the condensing vapor….
No, we know exactly how much water is evaporated in that example/supposition, We talk watts per square meter and we assumed one meter in a year, which is conservqative when talking about the ocean. But that gives you one cubic meter, 1000 liters, to evaoprate yearly divide that by seconds.
And I did not miss the point that this heat is released during condensation in the clouds. But that was not the issue, the issue was explicitely how much evaporation at ground level would reduce the warming of that same ground level.
The energy released into the clouds will warm the clouds resulting in additional radiant flux from the clouds down to earth and into outer space….
Perhaps it’s more accurate to state that the energy released into the clouds will slow down its cooling. If it would warm, the condensation would stop and hence the release of latent heat would too. The effect of the latent energy is the reason why the moist adiabatic lapse rate is less than the dry adiabatic lapse rate.
https://en.wikipedia.org/wiki/Lapse_rate
Dear Ben Dussan 15Jan19
“Evaporation of water takes a substantial amount of energy, which is no longer available for a temperature rise at the surface”
In case of evaporation of water at the surface it is clear that energy is withdrawn from its surroundings (surface and atmosphere close to the surface). This process is part of the convective processes that dictate the temperature laps rate on average 6,5K.km-1 in our troposphere. Models that use radiation transfer equations (RTE) under the assumption of local thermodynamic equilibrium (LTE) show a laps rate near surface of 36K.km-1 instead as the observed 6,5K.km-1.
“the net effect of additional water vapor, due to the temperature increase on account of doubling the CO2”
This is an important part of the most climate models. Wait for my full paper.
“Keep in mind that water evaporation can eventually result in an increase in cloud coverage and hence can result in a cooling effect. ….. by radiation….”
I fully agree.
“effect of overpopulation on the biosphere”
Please make clear in more detail what you mean.